Topological Photonics
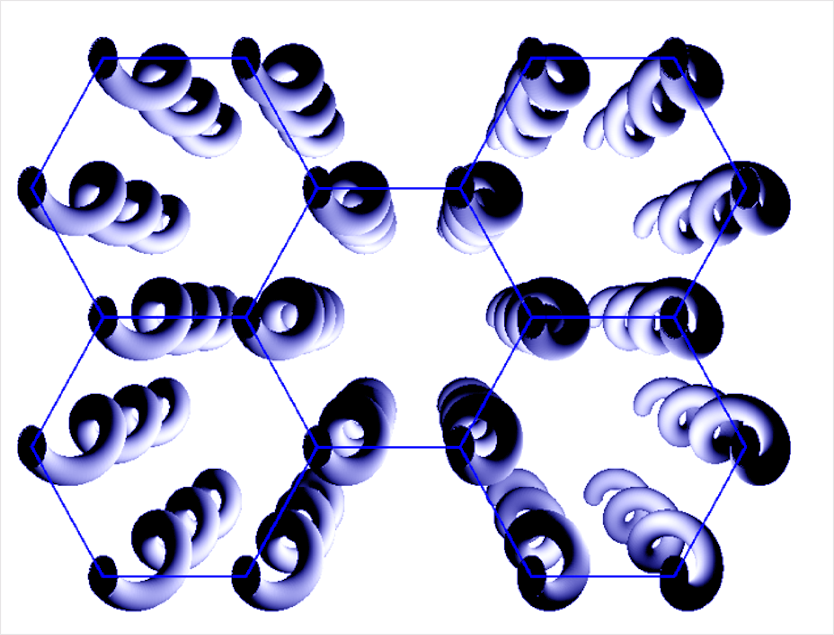
The most common degrees of freedom used for the description of a photonic system are frequency, wave vector, polarization and phase of the propagating light wave.
However, in the last few years a new degree of freedom entered photonics: Topology – a property of photonic materials that relates to the global structure of their frequency dispersions – has been emerging as another indispensable ingredient, opening a path forward to the discovery of fundamentally new states of light and possibly revolutionary applications, including photonic circuitry less dependent on isolators and slow light insensitive to disorder.
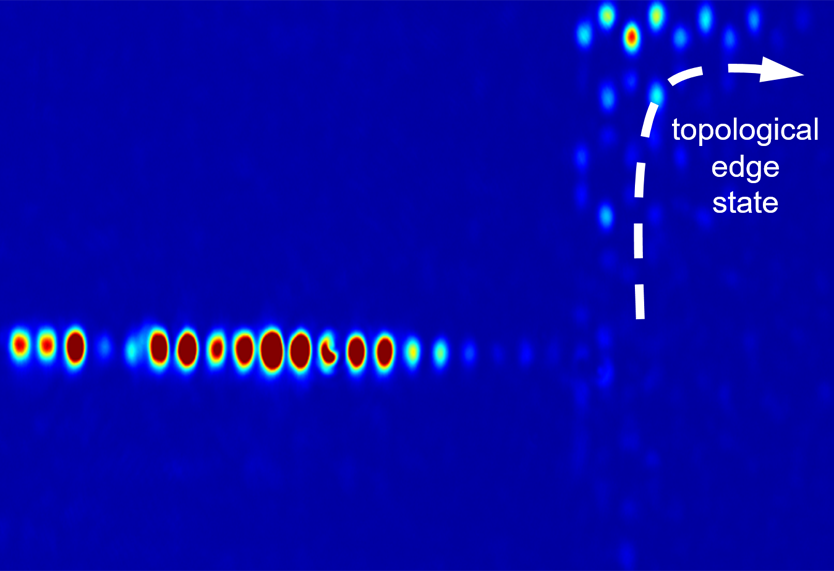
Based on laser-written photonic waveguide lattices [J. Phys. B 43, 163001 (2010)], we investigate the impact of topological quantities on the evolution of light. Recent results include the first demonstration of topological insulation of light [Nature 496, 196 (2013)], the first realization of demonstration of the topological creation and destruction of edge modes [Phys. Rev. Lett. 111, 103901 (2013)], in-band localized modes [Phys. Rev. Lett. 114, 245503 (2015)], the first demonstration of novel edge states in the graphene geometry [Nature Mater. 13, 57 (2014)], hopping kinks for arbitrary Z2-gauge transformations [Phys. Rev. Lett. 116(21), 213901 (2016)], the first demonstration of anomalous topological insulators [Nature Commun. 8, 13756 (2017)] and the first realization of a topological Anderson insulator [Nature 560, 461-465 (2018)], of a photonic topological insulator with fermionic time-reversal symmetry [Nature Mater. 19, 855–860 (2020)], and of a topological insulator with synthetic dimensions [Nature 567, 356 (2019)].
Notably, we expand our work also to the non-Hermitian regime [Phys. Rev. Lett. 113, 123903 (2014)], where we showed how to extract topological quantities from the evolution of a wave packet [Phys. Rev. Lett. 115, 040402 (2015)], and even the first PT-symmetric topological interface state [Nature Mater. 16, 433 (2017)]. Only recently we demonstrated a topological funnel for light [Science 368, 311 (2020)].
