Non-Hermitian Photonics
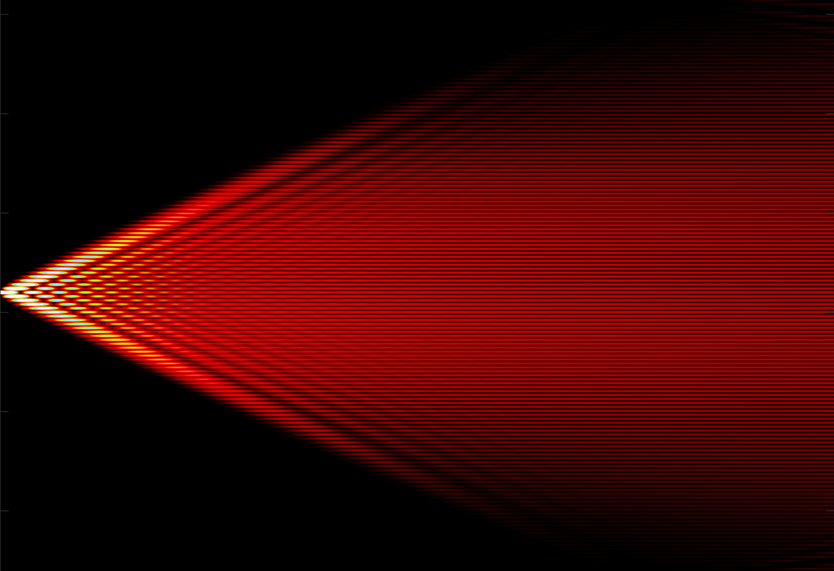
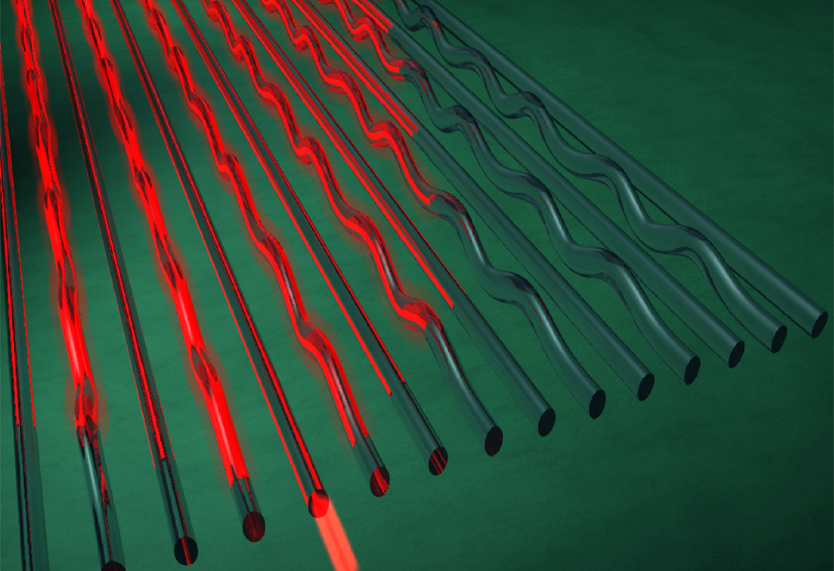
Parity-time (PT-) symmetric quantum mechanics is an extension of conventional Hermitian quantum mechanics into the complex domain. Importantly, PT-symmetry is not in conflict with the established mathematical framework, but rather a complex generalization of it. Originally considered only an interesting mathematical discovery with little or no hope of practical application, PT-symmetry has since been adapted to the field of photonics, where its implications as a radically new way to control light have spawned an area of intense theoretical and experimental research.
We contributed various results to this exciting field. First, we developed a thorough theory of so-called passive PT-symmetry [J. Opt. 6(16), 065501 (2014)], where no external pumping of the waveguides is required. These findings, combined with our expertise in femtosecond laser-written photonic lattices, enable the realization of entirely passive structures that nevertheless exhibit all the key features of PT symmetry. In this vein, we were able to demonstrate a peculiar effect exclusive to non-Hermitian systems: The abrupt transition from ballistic to diffusive transport of propagating wave packet after a characteristic evolution time [Nature Commun. 4, 2533 (2013)].
We also discovered that the PT-symmetric extension of two-dimensional graphene lattices can be used to generate the optical version of Tachyons [Phys. Rev. A 84, 021806(R) (2011)]. Another works were the experimental analysis of the impact of complex hopping between adjacent waveguides in a non-Hermitian lattice [Phys. Rev. Lett. 113, 123903 (2014)] and the realization of PT-symmetric flat band dispersion bands [Phys. Rev. Lett. 123, 183601 (2019)]. Coming full circle, we introduced PT-symmetry back to the quantum regime by analysing optical quantum random walks [New J. Phys. 15, 033008 (2013)] and observing non-hermitian quantum interference [Nature Photon. 13, 883 (2019)].
Currently, we are joining the fields of PT-symmetry and topological photonics. Three major breakthroughs along these lines were the first measurement of a topological invariant from a bulk measurement [Phys. Rev. Lett. 115, 040402 (2015)], the first realization of a two-dimensional topological PT-symmetric system [Nature Commun. 10, 435 (2019)], and the first observation of a topological PT-symmetric interface state [Nature Mater. 16, 433 (2017)]. Only recently we demonstrated a topological funnel for light [Science 368, 311 (2020)].
